RLC Parallel Resonant Circuit

Regarding the RLC Parallel Resonant Circuit, this article will explain the information below.

• What is RLC Parallel Resonant Circuit?
• "Impedance" and "Resonant Frequency" of RLC Parallel Resonant Circuit
• "Frequency Characteristics" and "Q factor" of RLC Parallel Resonant Circuit

What is RLC Parallel Resonant Circuit?

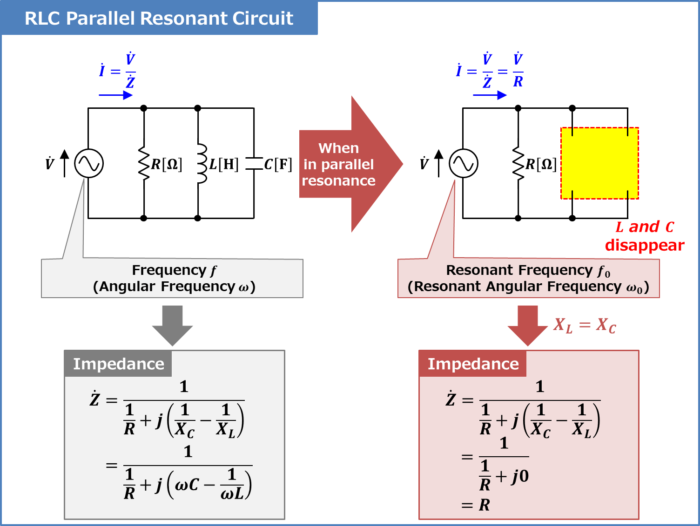
An RLC parallel resonant circuit is a circuit consisting of a resistor \(R\), an inductor \(L\), and a capacitor \(C\) connected in parallel.

A circuit in which an inductor \(L\) and a capacitor \(C\) are connected in parallel is called an RLC parallel resonant circuit because it resonates in parallel at some frequency. Some frequency is called resonant frequency. The symbol for the resonant frequency is often expressed as \(f_0\) or \(f_R\) (\(f_0\) is used in this article).

Then, in an RLC parallel resonant circuit, what is the state when the parallel resonance is at resonant frequency \(f_0\)?

In conclusion, when the RLC parallel resonant circuit is in parallel resonance at the resonant frequency \(f_0\), the reactance \(X_L={\omega}L\) of the inductor \(L\) and the reactance \(X_C=\displaystyle\frac{1}{{\omega}C}\) of the capacitor \(C\) cancel each other out (that is, \(X_L=X_C\)).

At the resonant frequency \(f_0\), the impedance of the RLC parallel resonant circuit is \({\dot{Z}}=R\) and the current flowing in the RLC parallel resonant circuit is \({\dot{I}}=\displaystyle\frac{V}{R}\).

Since the expression for the current flowing in the RLC parallel resonant circuit is \({\dot{I}}=\displaystyle\frac{V}{R}\), the inductor \(L\) and capacitor \(C\) disappear from the expression. In other words, at the resonant frequency \(f_0\), the inductor \(L\) and capacitor \(C\) are apparently eliminated, and only the resistor \(R\) is connected to the circuit.

Next, let us consider the above state from the impedance equation of the RLC parallel resonant circuit.

Impedance and Resonant Frequency of RLC Parallel Resonant Circuit

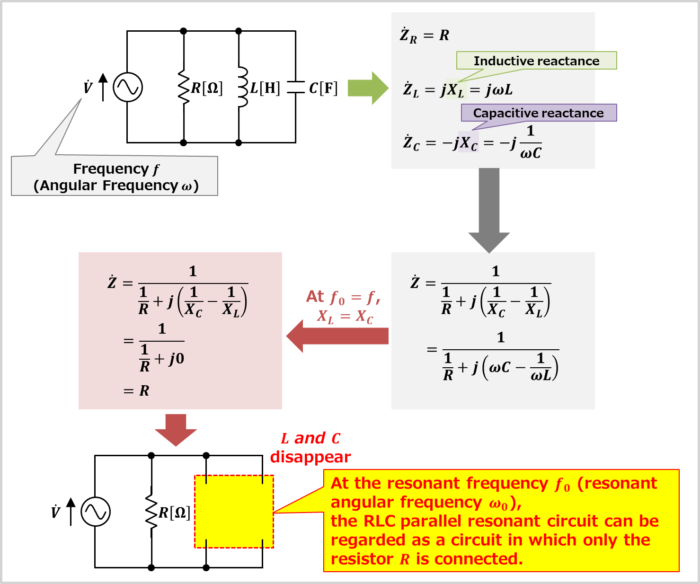
An RLC resonant parallel circuit is an electrical circuit consisting of a resistor \(R\), an inductor \(L\), and a capacitor \(C\) connected in parallel.

The impedance \({\dot{Z}}_R\) of the resistor \(R\), the impedance \({\dot{Z}}_L\) of the inductor \(L\), and the impedance \({\dot{Z}}_C\) of the capacitor \(C\) can be expressed by the following equations:

\begin{eqnarray}
{\dot{Z}}_R&=&R\tag{1}\\
\\
{\dot{Z}}_L&=&jX_L=j{\omega}L\tag{2}\\
\\
{\dot{Z}}_C&=&-jX_C=-j\frac{1}{{\omega}C}=\frac{1}{j{\omega}C}\tag{3}
\end{eqnarray}

, where \({\omega}\) is the angular frequency, which is equal to \(2{\pi}f\), and \(X_L\) is called inductive reactance, which is the resistive component of inductor \(L\) and \(X_C\) is called capacitive reactance, which is the resistive component of capacitor \(C\).

The sum of the reciprocals of each impedance is the reciprocal of the impedance \({\dot{Z}}\) of the RLC parallel circuit. Therefore, it can be expressed by the following equation.

\begin{eqnarray}
\frac{1}{{\dot{Z}}}&=&\frac{1}{{\dot{Z}_R}}+\frac{1}{{\dot{Z}_L}}+\frac{1}{{\dot{Z}_C}}\\
\\
&=&\frac{1}{R}+\frac{1}{jX_L}+\frac{1}{-jX_C}\\
\\
&=&\frac{1}{R}+j\left(\frac{1}{X_C}-\frac{1}{X_L}\right)\\
\\
&=&\frac{1}{R}+j\left(\frac{1}{\displaystyle\frac{1}{{\omega}C}}-j\frac{1}{{\omega}L}\right)\\
\\
&=&\frac{1}{R}+j\left({\omega}C-\frac{1}{{\omega}L}\right)\tag{4}
\end{eqnarray}

From equation (4), by interchanging the denominator and numerator, the following equation is obtained:

\begin{eqnarray}
{\dot{Z}}=\frac{1}{\displaystyle\frac{1}{R}+j\left(\displaystyle\frac{1}{X_C}-\displaystyle\frac{1}{X_L}\right)}=\frac{1}{\displaystyle\frac{1}{R}+j\left({\omega}C-\displaystyle\frac{1}{{\omega}L}\right)}\tag{5}
\end{eqnarray}

The magnitude \(Z\) of the impedance of the RLC parallel resonant circuit is expressed by the following equation.

\begin{eqnarray}
Z=|{\dot{Z}}|&=&\frac{1}{\sqrt{\left(\displaystyle\frac{1}{R}\right)^2+\left(\displaystyle\frac{1}{X_C}-\displaystyle\frac{1}{X_L}\right)^2}}\\
\\
&=&\frac{1}{\sqrt{\left(\displaystyle\frac{1}{R}\right)^2+\left({\omega}C-\displaystyle\frac{1}{{\omega}L}\right)^2}}\tag{6}
\end{eqnarray}

The RLC parallel resonant circuit is in parallel resonance when the reactance \(X_L={\omega}L\) of the inductor \(L\) and the reactance \(X_C=\displaystyle\frac{1}{{\omega}C}\) of the capacitor \(C\) cancel each other (i.e., when \(X_L=X_C\)). If the angular frequency \({\omega}\) at \(X_L=X_C\) is the resonant angular frequency \({\omega}_0\), the following equation holds.

\begin{eqnarray}
X_L&=&X_C\\
\\
{\Leftrightarrow}{{\omega}_0}L&=&\frac{1}{{{\omega}_0}C}\tag{7}\\
\\
{\Leftrightarrow}{{\omega}_0}C-\frac{1}{{{\omega}_0}L}&=&0\tag{8}
\end{eqnarray}

Using equation (7), the resonant angular frequency \({\omega}_0\) and resonant frequency \(f_0\) of the RLC parallel resonant circuit can be obtained as follows.

\begin{eqnarray}
{{\omega}_0}L&=&\frac{1}{{{\omega}_0}C}\\
\\
{\Leftrightarrow}{{\omega}_0}^2LC&=&1\\
\\
{\Leftrightarrow}{\omega}_0&=&\frac{1}{\sqrt{LC}}\tag{9}\\
\\
{\Leftrightarrow}f_0&=&\frac{1}{2{\pi}\sqrt{LC}}\tag{10}
\end{eqnarray}

From equations (9) and (10), it can be seen that the resonant angular frequency \({\omega}_0\) and resonant frequency \(f_0\) of the RLC parallel resonant circuit are determined by the inductor \(L\) and capacitor \(C\), and the resistance value \(R\) of the resistor is irrelevant.

Using equation (8), the impedance \({\dot{Z}}\) of the RLC parallel resonant circuit and its magnitude \(Z\) at the resonant frequency \(f_0\) (resonant angular frequency \({\omega}_0\)) are as follows.

\begin{eqnarray}
{\dot{Z}}&=&\frac{1}{\displaystyle\frac{1}{R}+j\left({\omega}_0C-\displaystyle\frac{1}{{\omega}_0L}\right)}=\frac{1}{\displaystyle\frac{1}{R}+j0}=\frac{1}{\displaystyle\frac{1}{R}}=R\tag{11}\\
\\
Z&=&\frac{1}{\sqrt{\left(\displaystyle\frac{1}{R}\right)^2+\left({\omega}_0C-\displaystyle\frac{1}{{\omega}_0L}\right)^2}}=\frac{1}{\sqrt{\left(\displaystyle\frac{1}{R}\right)^2+0^2}}=\frac{1}{\sqrt{\left(\displaystyle\frac{1}{R}\right)^2}}=R\tag{12}
\end{eqnarray}

Let us look at equations (11) and (12). At the resonant frequency \(f_0\) (resonant angular frequency \({\omega}_0\)), the inductor \(L\) and capacitor \(C\) disappear from the impedance equation of the RLC parallel resonant circuit.

In other words, at the resonant frequency \(f_0\) (resonant angular frequency \({\omega}_0\)), the RLC parallel resonant circuit can be regarded as a circuit in which the inductor \(L\) and capacitor \(C\) disappear and only the resistor \(R\) is connected.

Q Factor of RLC Parallel Resonant Circuit

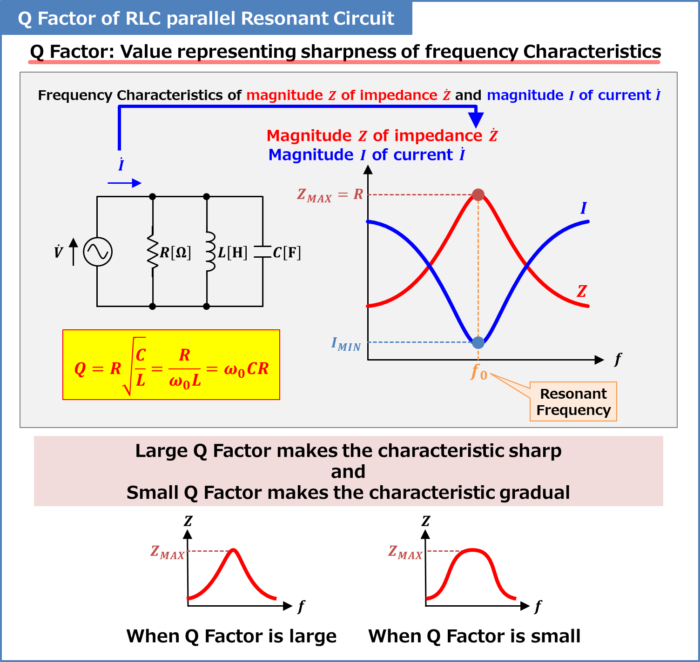
The frequency Characteristics of the magnitude \(Z\) of the impedance of the RLC parallel resonant circuit expressed in equation (5) is shown in the figure above.

As can be seen from the frequency characteristics, the magnitude \(Z\) of the impedance of the RLC parallel resonant circuit has a maximum value \(Z_{MAX}=R\) at the resonant frequency \(f_0\) and increases as the resonant frequency moves away from \(f_0\).

Therefore, the magnitude \(I\) of the current flowing in the RLC parallel resonant circuit at the resonant frequency \(f_0\) is the minimum value \(I_{MIN}\), and \(I_{MIN}\) is expressed by the following equation.

\begin{eqnarray}
I_{MIN}&=&\frac{V}{Z_{MAX}}\\
\\
&=&\frac{V}{R}\tag{13}
\end{eqnarray}

Resonant circuits also have a value called the Q factor. The larger the Q factor, the sharper the frequency response, and the smaller the Q factor, the more gradual the response.

In the case of an RLC parallel resonant circuit, the Q factor is expressed by the following equation.

\begin{eqnarray}
Q=R\sqrt{\frac{C}{L}}=\frac{R}{{\omega}_0L}={\omega}_0CR\tag{14}
\end{eqnarray}

The larger the resistance of the resistor \(R\), the smaller the inductance of the inductor \(L\), and the larger the capacitance of the capacitor \(C\), the larger the Q factor, resulting in a sharper frequency response.

Summary

This article described the following information about "RLC Parallel Resonant Circuit".

1. What is RLC Parallel Resonant Circuit?
2. "Impedance" and "Resonant Frequency" of RLC Parallel Resonant Circuit
3. "Frequency Characteristics" and "Q factor" of RLC Parallel Resonant Circuit

Thank you for reading.