Q Factor of RLC Parallel Resonant Circuit

Regarding the Q Factor of RLC Parallel Resonant Circuit, this article will explain the information below.

• What is the Q factor of RLC parallel resonant circuit?
• Derivation of Q factor of RLC parallel resonant circuit
• Relationship between "Q factor" and "current flowing through the inductor L and capacitor C

What is the Q factor of RLC parallel resonant circuit?

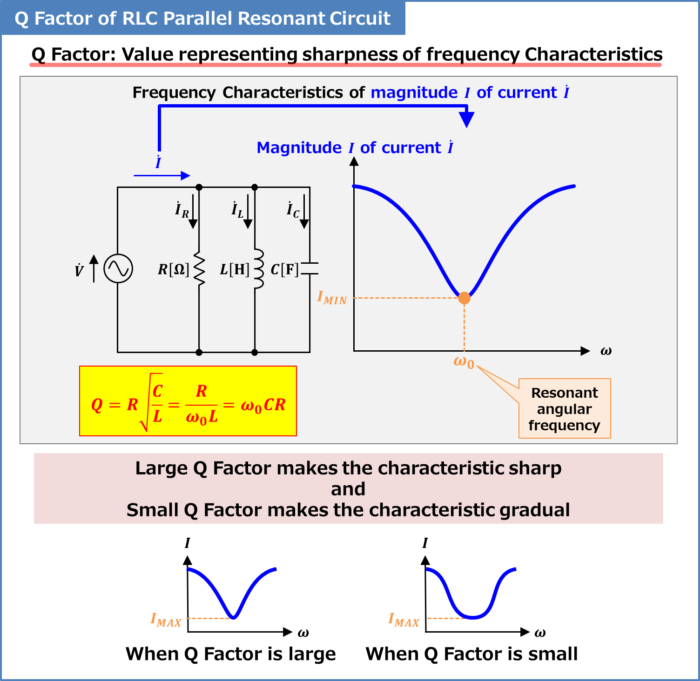
Q factor is a value that expresses the sharpness of the frequency characteristics. A large Q factor makes the frequency characteristics sharper, while a small Q factor makes the frequency characteristics more gradual.

The above figure shows the frequency characteristics of the magnitude \(I\) of the current flowing in an RLC parallel resonant circuit. The RLC parallel resonant circuit is a circuit consisting of a resistor \(R\), an inductor \(L\), and a capacitor \(C\) connected in parallel, and the magnitude \(I\) of current reaches its minimum value \(I_{MIN}\) at the angular frequency \({\omega}_0\left(=\displaystyle\frac{1}{\sqrt{LC}}\right)\) where the inductor \(L\) and capacitor \(C\) resonate.

In an RLC parallel resonant circuit, the Q factor is expressed by the following equation (the derivation of the following equation will be explained later).

In the above equation, \({\omega}_0\) is the resonant angular frequency, \(R\) is the resistance of the resistor, \(L\) is the inductance of the inductor, and \(C\) is the capacitance of the capacitor.

From equation (1), the smaller \({\Delta}{\omega}(={\omega}_2-{\omega}_1)\) (the sharper the frequency response), the larger the Q factor.

Derivation of Q factor of RLC parallel resonant circuit

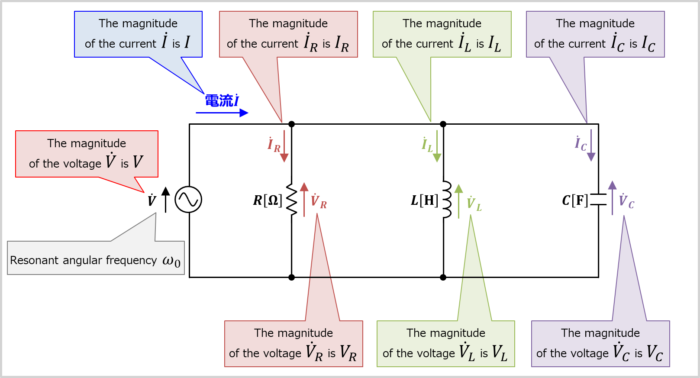
The RLC resonant parallel circuit is an electrical circuit consisting of a resistor \(R\), an inductor \(L\), and a capacitor \(C\) connected in parallel.

When the resistance of resistor \(R\) is \(R{\mathrm{[{\Omega}]}}\), the inductance of inductor \(L\) is \(L{\mathrm{[H]}}\), and the capacitance of capacitor \(C\) is \(C{\mathrm{[F]}}\), the impedance \({\dot{Z}}\) of the RLC parallel resonant circuit becomes the following equation.

The definition of Q factor in an RLC parallel resonant circuit is the ratio of "the magnitude \(I_L\) of the current flowing in the inductor \(L\) (or the magnitude \(I_C\) of the current flowing in the capacitor \(C\)) at resonance" and "the magnitude \(I_R\) of the current flowing in the resistor \(R\) at resonance".

If this definition is written in an equation, the Q factor of the RLC parallel resonance circuit is expressed by the following equation.

\begin{eqnarray}
Q=\frac{I_L}{I_R}=\frac{I_C}{I_R}\tag{2}
\end{eqnarray}

Now, let's find "the magnitude \(I_R\) of the current flowing in the resistor \(R\)", "the magnitude \(I_L\) of the current flowing in the inductor \(L\)", and "the magnitude \(I_C\) of the current flowing in the capacitor \(C\)" at the resonance angular frequency \({\omega}_0\).

• The magnitude \(I_R\) of the current flowing in the resistor \(R\)
  • Since the magnitude \(V_R\) of the voltage across the resistor \(R\) is equal to the magnitude \(V\) of the power supply voltage, the magnitude \(I_R\) of the current flowing through the resistor \(R\) is expressed by the following equation.
    \begin{eqnarray}
    I_R=\frac{V_R}{R}=\frac{V}{R}\tag{3}
    \end{eqnarray}
• The magnitude \(I_L\) of the current flowing in the inductor \(L\)
  • The magnitude \(V_L\) of the voltage across the inductor \(L\) is equal to the magnitude \(V\) of the power supply voltage. The magnitude \(X_L\) of the reactance of inductor \(L\) is expressed by "\(X_L={\omega}L\)". Therefore, the magnitude \(I_L\) of the current flowing through the inductor \(L\) at the resonant angular frequency \({\omega}_0\) is expressed by the following equation.
    \begin{eqnarray}
    I_L=\frac{V_L}{X_L}=\frac{V}{{\omega}_0L}\tag{4}
    \end{eqnarray}
• The magnitude \(I_C\) of the current flowing in the capacitor \(C\)
  • The magnitude \(V_C\) of the voltage across the capacitor \(C\) is equal to the magnitude \(V\) of the power supply voltage. The magnitude \(X_C\) of the reactance of capacitor \(C\) is expressed by "\(X_C=\displaystyle\frac{1}{{\omega}C}\)". Therefore, the magnitude \(I_C\) of the current flowing through the capacitor \(C\) at the resonant angular frequency \({\omega}_0\) is expressed by the following equation.
    \begin{eqnarray}
    I_C=\frac{V_C}{X_C}=\frac{V}{\displaystyle\frac{1}{{\omega}_0C}}={\omega}_0CV\tag{5}
    \end{eqnarray}

From equations (2)-(5), the Q factor of the RLC parallel resonant circuit can be expressed by the following equation.

\begin{eqnarray}
Q&=&\frac{I_L}{I_R}=\frac{\displaystyle\frac{V}{{\omega}_0L}}{\displaystyle\frac{V}{R}}=\frac{R}{{\omega}_0L}\tag{6}\\
\\
Q&=&\frac{I_C}{I_R}=\frac{{\omega}_0CV}{\displaystyle\frac{V}{R}}={\omega}_0CR\tag{7}\\
\end{eqnarray}

In addition, using "\({\omega}_0=\displaystyle\frac{1}{\sqrt{LC}}\)" in equations (6) or (7), the following equation is obtained.

\begin{eqnarray}
Q=R\sqrt{\frac{C}{L}}\tag{8}
\end{eqnarray}

This concludes the derivation of the Q factor of the RLC parallel resonant circuit.

Relationship between "Q factor" and "current flowing through the inductor L and capacitor C

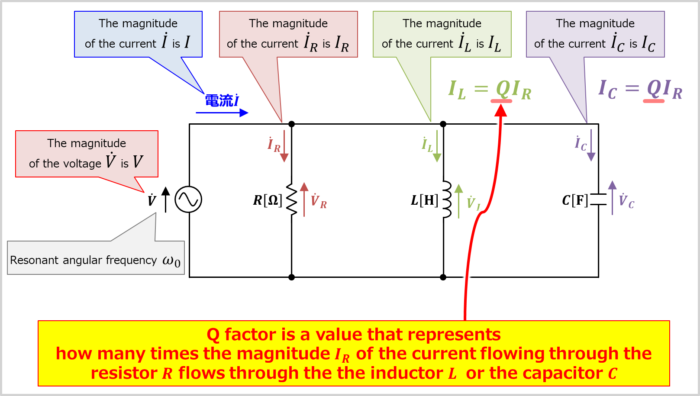
From equation (6), the magnitude \(I_L\) of the current flowing in the inductor \(L\) at the resonant angular frequency \({\omega}_0\) can be expressed by the following equation

\begin{eqnarray}
I_L=Q×I_R\tag{9}
\end{eqnarray}

Similarly, the magnitude \(I_C\) of the current flowing through the capacitor \(C\) is given by the following equation.

\begin{eqnarray}
I_C=Q×I_R\tag{10}
\end{eqnarray}

Therefore, the Q factor is also a value that expresses how many times the magnitude \(I_R\) of the current flowing through the resistor \(R\) flows through the inductor \(L\) or the capacitor \(C\). The larger the Q factor, the larger the current flowing through the inductor \(L\) and the capacitor \(C\).

I will now explain the RLC parallel resonance circuit in detail. At the beginning of this section, we explained that "at the angular frequency \({\omega}_0\left(=\displaystyle\frac{1}{\sqrt{LC}}\right)\) where the inductor \(L\) and the capacitor \(C\) resonate, the magnitude of the current \(I\) flowing in the RLC parallel resonance circuit reaches the minimum value \(I_{MIN}\). Let us derive this value of \(I_{MIN}\).

The admittance \({\dot{Y}}\) of an RLC parallel resonant circuit is expressed by the following equation.

\begin{eqnarray}
{\dot{Y}}&=&\frac{1}{R}+\frac{1}{j{\omega}L}+j{\omega}C\\
\\
&=&\frac{1}{R}+j\left({\omega}C-\frac{1}{{\omega}L}\right)\tag{11}
\end{eqnarray}

, where \({\omega}\) is the angular frequency, which is equal to \(2{\pi}f\).

The magnitude \(Y\) of the admittance of the RLC parallel resonant circuit is expressed by the following equation.

\begin{eqnarray}
Y=|{\dot{Y}}|=\sqrt{\left(\frac{1}{R}\right)^2+\left({\omega}C-\displaystyle\frac{1}{{\omega}L}\right)^2}\tag{12}
\end{eqnarray}

Therefore, when the magnitude of the supply voltage is \(V\), the magnitude \(I\) of the current flowing in the RLC parallel resonant circuit is expressed by the following equation.

\begin{eqnarray}
I=VY=V\sqrt{\left(\frac{1}{R}\right)^2+\left({\omega}C-\displaystyle\frac{1}{{\omega}L}\right)^2}\tag{13}
\end{eqnarray}

At the angular frequency (resonant angular frequency) )\({\omega}_0\left(=\displaystyle\frac{1}{\sqrt{LC}}\right)\), the inductor \(L\) and the capacitor \(C\) are in resonance, and the following equation holds.

\begin{eqnarray}
{\omega}_0&=&\frac{1}{\sqrt{LC}}\\
\\
{\Leftrightarrow}{{\omega}_0}^2&=&\frac{1}{LC}\\
\\
{\Leftrightarrow}{{\omega}_0}L&=&\frac{1}{{{\omega}_0}C}\\
\\
{\Leftrightarrow}{\omega}_0C-\displaystyle\frac{1}{{\omega}_0L}&=&0\tag{14}
\end{eqnarray}

At resonant angular frequency \({\omega}_0\), the magnitude \(I\) of the current flowing in the RLC parallel circuit is the minimum value \(I_{MIN}\). \(I_{MIN}\) is expressed by the following equation.

\begin{eqnarray}
I_{MIN}&=&V\sqrt{\left(\frac{1}{R}\right)^2+\left({\omega}_0C-\displaystyle\frac{1}{{\omega}_0L}\right)^2}\\
\\
&=&V\sqrt{\left(\frac{1}{R}\right)^2+0^2}\\
\\
&=&\frac{V}{R}\tag{15}
\end{eqnarray}

If we look at equations (3) and (15), we see that \(I_R\) and \(I_{MIN}\) have the same value. In other words, equations (9) and (10) can be transformed into the following equation

\begin{eqnarray}
I_L&=&Q×I_R=Q×I_{MIN}\tag{16}\\
\\
I_C&=&Q×I_R=Q×I_{MIN}\tag{17}
\end{eqnarray}

Therefore, the Q factor is also a value that expresses how many times the magnitude \(I_{MIN}\) of the current flowing from the power supply flows through the inductor \(L\) or capacitor \(L\) at resonance (at the resonance angular frequency \({\omega}_0\)). The larger the Q factor, the larger the current flowing through the inductor \(L\) and capacitor \(C\).

Summary

This article described the following information about "Q Factor of RLC Parallel Resonant Circuit".

1. What is the Q factor of RLC parallel resonant circuit?
2. Derivation of Q factor of RLC parallel resonant circuit
3. Relationship between "Q factor" and "current flowing through the inductor L and capacitor C

Thank you for reading.